Serendip is an independent site partnering with faculty at multiple colleges and universities around the world. Happy exploring!
The Three Doors of Serendip: Itemizing
|
The Three Doors of Serendip:
Itemizing
|
Door images from Woodstone |
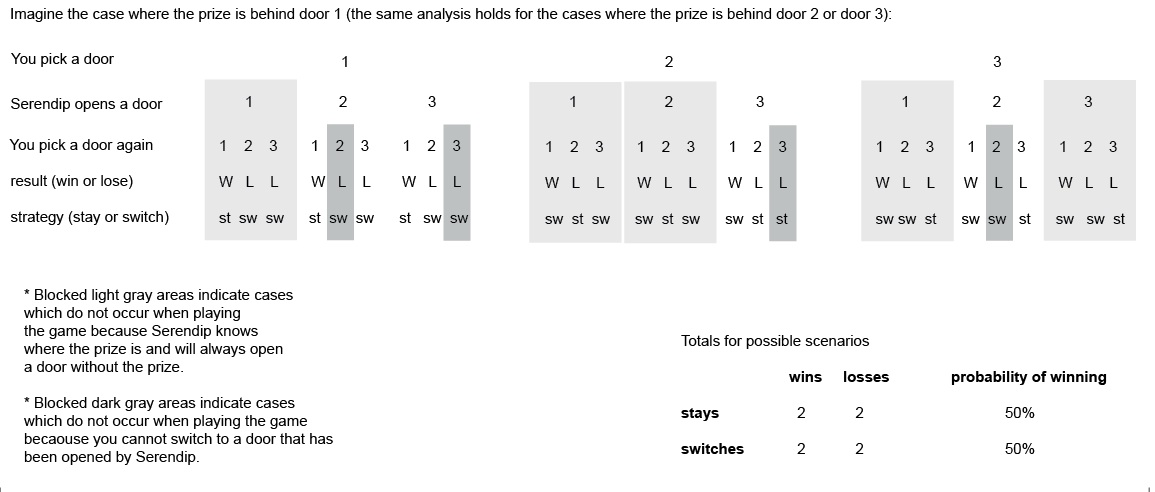
Itemizing, in cases where it's possible, may seem like the most "logical" way to reach a broader understanding. And sometimes it may. The table below shows a solution to the Three Door Problem reached by trying to list all possible cases and their outcomes. As you'll see, the itemization in this case yields the same conclusion that is observed empirically.
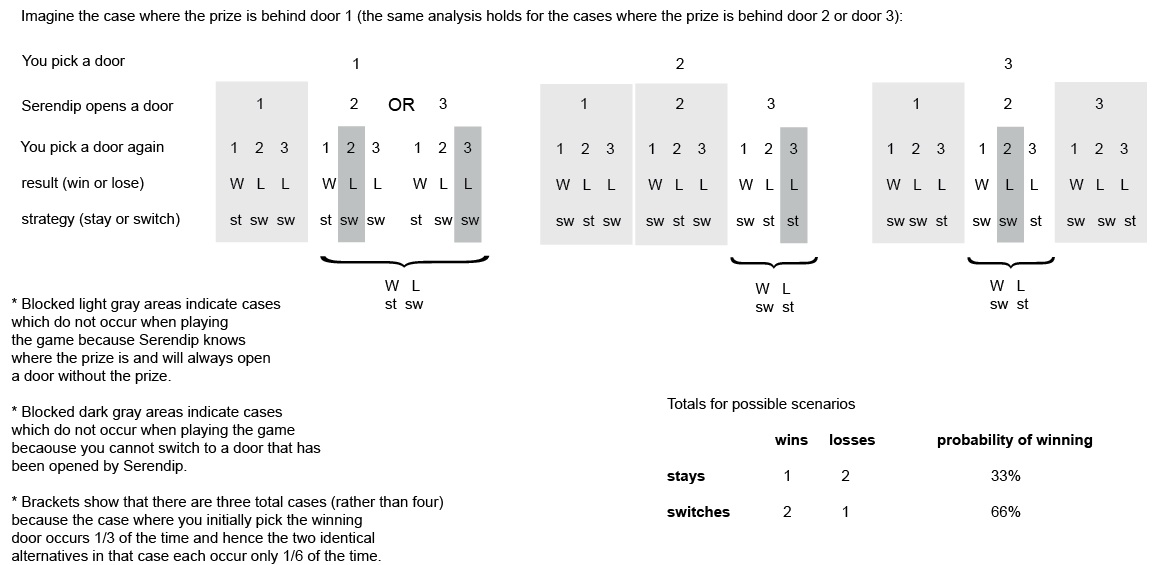
Below is an itemization like the one above, that does match the empirical conclusion because it has been modified to reflect the relative number of different times that different cases occur randomly. Would one know without the empirical observations that this modification is needed as a part of the itemization process?
An additional problem with itemization is that it may or may not actually yield a sense of broader understanding (see The Four Color Problem). To put it differently, seeking broader understanding is seeking a sense of absence of conflict between various kinds of understanding and this may or may not result from itemization, even when it matches empirical observations.
|
Hands on understanding |
Experimental understanding |

Broader understanding |





Comments
Post new comment